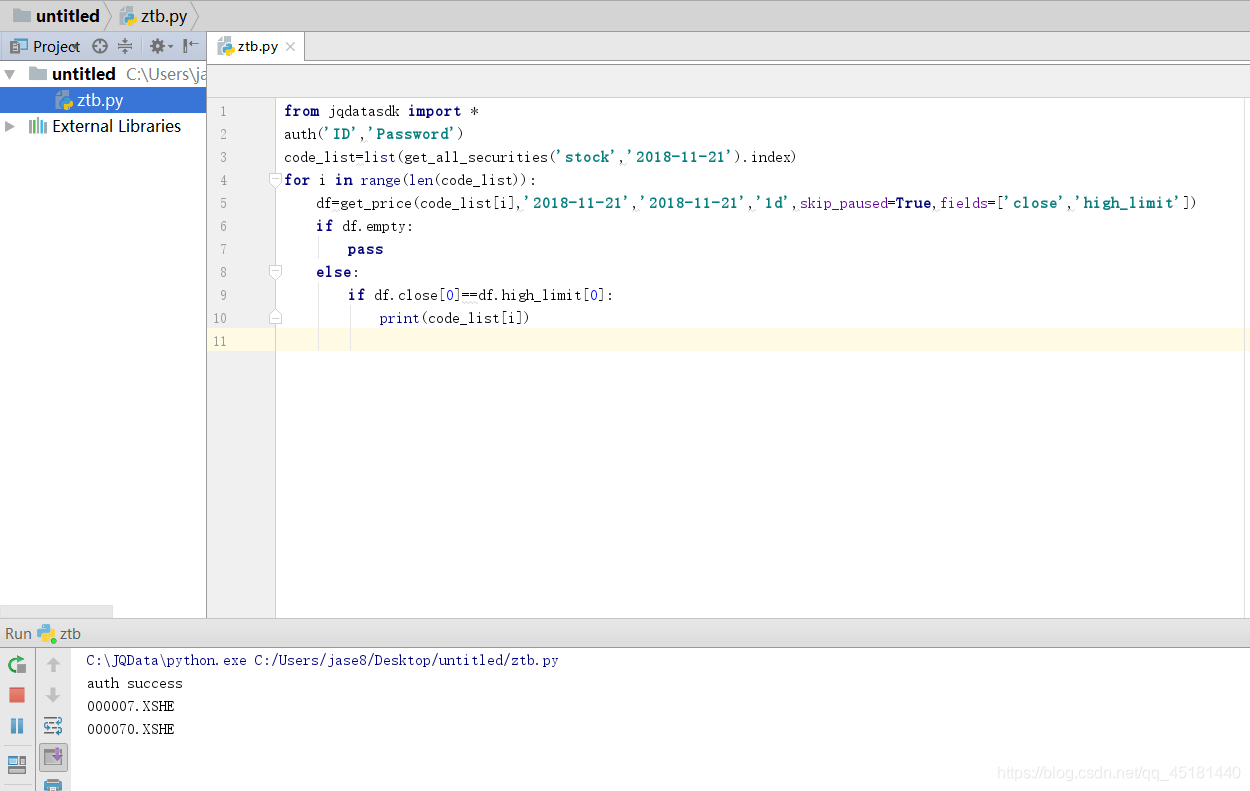
练习5: 函数和代码复用
1 .1实例7:七段数码管绘制
描述
这是"实例"题,与课上讲解实例相同,请作答检验学习效果。
七段数码管是一种展示数字的有效方式。
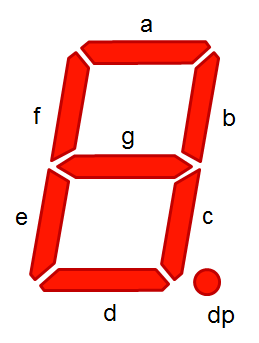
请用程序绘制当前系统时间对应的七段数码管,效果如下:

要求如下:
(1) 使用 time库获得系统当前时间,格式如下:20190411
(2) 绘制对应的七段数码管
(3) 数码管风格不限
请在本机编写代码完成实例,建议有趣的风格请在Python123的绘图专区上传展示。
【参考代码】
import turtle as t
import time
def drawGap(): #绘制数码管间隔t.penup()t.fd(5)
def drawLine(draw): #绘制单段数码管drawGap()t.pendown() if draw else t.penup()t.fd(40)drawGap()t.right(90)
def drawDigit(d): #根据数字绘制七段数码管drawLine(True) if d in [2,3,4,5,6,8,9] else drawLine(False)drawLine(True) if d in [0,1,3,4,5,6,7,8,9] else drawLine(False)drawLine(True) if d in [0,2,3,5,6,8,9] else drawLine(False)drawLine(True) if d in [0,2,6,8] else drawLine(False)t.left(90)drawLine(True) if d in [0,4,5,6,8,9] else drawLine(False)drawLine(True) if d in [0,2,3,5,6,7,8,9] else drawLine(False)drawLine(True) if d in [0,1,2,3,4,7,8,9] else drawLine(False)t.left(180)t.penup()t.fd(20)
def drawDate(date):t.pencolor("red")for i in date:drawDigit(eval(i))
def main():t.setup(800, 350, 200, 200)t.penup()t.fd(-300)t.pensize(5)drawDate(time.strftime('%Y%m%d',time.gmtime()))t.done()
main()
基本思路:
步骤 1:绘制单个数字对应的码管步骤 2:获得当前系统时间,变成字符串,绘制对应的码管思维方法:
-模块化思维:确定接口,封装功能-规则化思维:抽象过程为规则,计算机自动执行-化繁为简:将大功能变小组合,分而治之
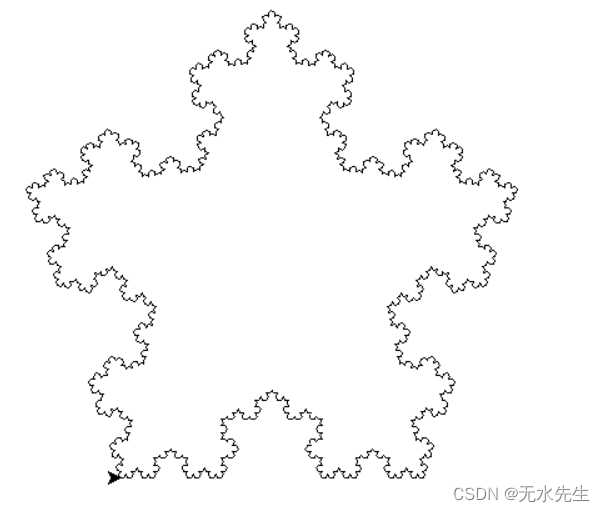
1.2实例8:科赫雪花小包裹
描述
这是"实例"题,与课上讲解实例相同,请作答检验学习效果。
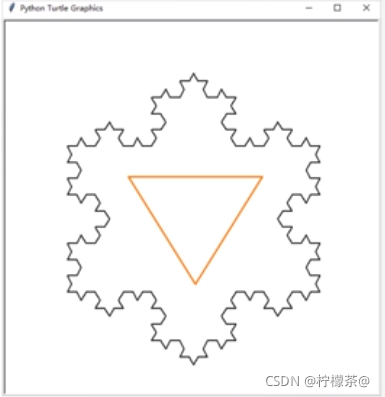
科赫曲线,也叫雪花曲线。绘制科赫曲线。
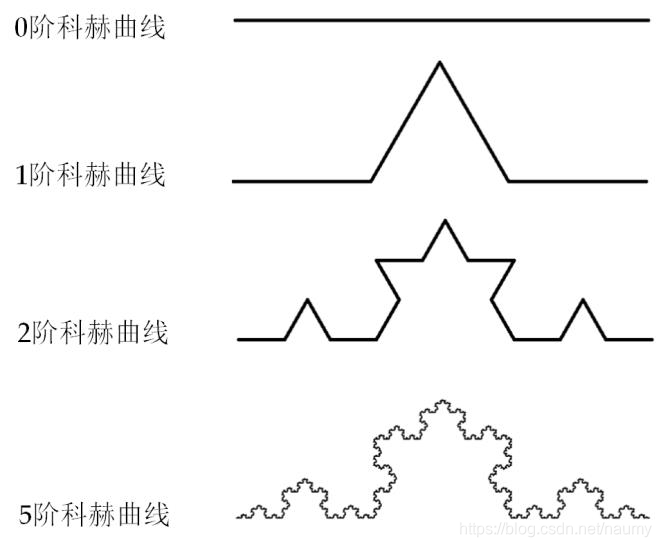
请补充编程模板中代码,完成功能:获得用户输入的整数N,作为阶,绘制N阶科赫曲线。
【参考代码】
import turtle
def koch(size, n):if n == 0:turtle.fd(size)else:for angle in [0, 60, -120, 60]:turtle.left(angle)koch(size/3, n-1)def main(level):turtle.setup(600,600)turtle.penup()turtle.goto(-200, 100)turtle.pendown()turtle.pensize(2)koch(400,level) turtle.right(120)koch(400,level)turtle.right(120)koch(400,level)turtle.hideturtle()try:level = eval(input("请输入科赫曲线的阶: "))main(level)
except:print("输入错误")
(1) 基本思路:
-递归思想:函数 +分支
-递归链条:线段的组合
-递归基例:初始线段(2) 分形几何是一种迭代的图,广泛存在于自然界中,请尝试选择一个新曲线绘制:
-康托尔集、谢宾斯基三角形门格海绵 …
-龙形曲线 、空间填充科赫…
-函数递归的深入应用 …
1.3任意累积
描述
请根据编程模板补充代码,计算任意个输入数字的乘积。
注意,仅需要在标注…的地方补充一行或多行代码。
| 输入 | 输出 |
|---|---|
| 1,2,3,4 | 24 |
参考代码如下:
def cmul(a, *b):m = afor i in b:m *= ireturn mprint(eval("cmul({})".format(input())))
该程序需要注意两个内容:
无限制数量函数定义的方法,其中b在函数cmul中表达除了a之外的所有输入参数;
以字符串形式调用函数的方法,"cmul()"与eval()的组合,提供了很多灵活性。
1.4斐波那契数列计算
描述
根据编程模板补充代码,计算斐波那契数列的值,具体功能如下:
1. 获取用户输入整数N,其中,N为正整数
2. 计算斐波那契数列的值
如果将斐波那契数列表示为fbi(N),对于整数N,值如下:
fbi(1)和fbi(2)的值是1,当N>2时,fbi(N) = fbi(N-1) + fbi(N-2)
请采用递归方式编写。
| 输入 | 输出 |
|---|---|
| 4 | 3 |
【参考代码】
def fbi(n):if n == 1 or n == 2:return 1 else:return fbi(n-1) + fbi(n-2)n = eval(input())
print(fbi(n))
基本的递归使用,不解释。
1.5汉诺塔实践
描述
汉诺塔问题大家都清楚,这里不再赘述。
请补充编程模板中代码,完成如下功能:
有三个圆柱A、B、C,初始时A上有N个圆盘,N由用户输入给出,最终移动到圆柱C上。
每次移动步骤的表达方式示例如下:[STEP 10] A->C。其中,STEP是步骤序号,宽度为4个字符,右对齐。
请编写代码,获得输入N后,输出汉诺塔移动的步骤。
输入格式
一个整数
输出格式
每个步骤一行,每行参考格式如下:[STEP 10] A->C
| 输入 | 输出 |
|---|---|
| 3 | [STEP 1] A->C [STEP 2] A->B [STEP 3] C->B [STEP 4] A->C [STEP 5] B->A [STEP 6] B->C [STEP 7] A->C |
【参考代码】
steps = 0
def hanoi(src, des, mid, n):global stepsif n == 1:steps += 1print("[STEP{:>4}] {}->{}".format(steps, src, des))else:hanoi(src, mid, des, n-1)steps += 1print("[STEP{:>4}] {}->{}".format(steps, src, des)) hanoi(mid, des, src, n-1)
N = eval(input())
hanoi("A", "C", "B", N)
汉诺塔实例十分经典,学习每门语言都要写一遍。
这个例子要注意:全局变量的使用以及递归的用法。递归用法注意:函数定义+分支表示。
python使用函数作为参数
在实际使用中,我们有时希望将函数作为参数传递给另一个方法使用。
import timedef loop():is_ok = Truefor i in range(3):print("i", i)if is_ok:breakelse:continue# python中函数作为参数传递 使用函数当做入参
def run(func):print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))func()print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))def test():print("我只是个测试文件")# 使用函数当做入参,函数本身包含参数
def run(func, a, b):print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))func(a, b)print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))def plus(a, b):print(a + b)# 使用函数当做入参,函数本身包含不确定个数的入参
def run(func, a, *args):print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))func(*args)print(time.strftime('%Y/%M/%D %H%M%S', time.localtime(time.time())))def plus(*args):temp = 0for i in args:trmp =temp + iprint(temp)if __name__ == '__main__':loop()run(test)run(plus, 3, 5)run(plus, 5, 3, 5, 8)